Cracking the Code of The World: Mathematics
“Mathematics is, in its way, the poetry of logical ideas.”
“Mathematics is, in its way, the poetry of logical ideas.”
-Albert Einstein
Mathematics certainly takes up an undeserved reputation in school— coined ‘too boring’, ‘frustrating’ or even ‘frightening’. However, its significance transcends beyond the classroom, being the core foundation of logic. Without realising, all of our actions that we perform daily can be quantified using mathematics, which we often do without even thinking.
Mathematics in the Beginning: A Brief History
One of the first discoveries of Mathematics dates back to at least 30,000 B.C, where very early examples of counting are shown. Archimedes — regarded as one of the most remarkable Greek mathematicians and also widely known as the father of mathematics, described mathematics as ‘perspire’; a Latin word for perspective defined as “learning” in Greek whose root in Hebrew is “thinking.” Fascinating as it might be, the lateral portrayals of mathematics gives us a clear picture of the role of crucial and logical thinking in any field of endeavour.
All of mathematics is undoubtedly interconnected with one another: algebra, geometry, analysis — you name it. These concepts are helpful for applications in other mathematics notions and closely related fields. Of course, not every piece of the puzzle will be used all of the time; their purpose can be cast over a wide set of equations.
Crossing Roads with Maths
Let’s give an example of everyday life where an understanding of mathematics comes in handy. Imagine yourself standing on the side of a busy road, waiting to cross the street. Before taking your first step, you will check both sides to see whether nearby vehicles are approaching. Now the remarkable thing starts happening because, without even realising it, very complex mathematical calculations begin to run through your head. You need to evaluate the following criteria in fractions of a second:
The velocity and acceleration of the incoming vehicles and the time it reaches your position.
The speed you can cross the road.
The width of road and time to cover the distance.
The cars arrive at the rate λ. The time needed for you to cross the road safely is denoted as k. You will have to wait until you see a gap of time at least k between the coming cars. If the gap between the car that has just passed and the next one is at least k, you begin to cross the road. Let T denote the random time you need to wait by the road. What is the expected time you need to cross this particular road?
This question could be open to interpretation, but to keep it simple, reading it as when the pedestrian arrives at the road, he can immediately see the street from where he stands to 𝑘 time units along the road so that he can identify a coming time gap of 𝑘 units. Accordingly, if this section of road is empty at that time, he crosses immediately so that 𝑇=0 and the total time taken for the crossing is 𝑇+𝑘=𝑘. Let 𝑋 be the time of the first car’s passing and the condition of the event 𝑋<𝑘, meaning that the following vehicle lies in that 𝑘-length section of the road:
A simple road-crossing action involves extensive evaluation of mathematical concepts happening so quickly without us noticing it. This is one of the few millions of illustrations where mathematics is applied in our life. Mathematics emerged as a means of solving problems and later became the language of other sciences. The symmetry of the world is discovered by mathematics as we see it today.
All About Numbers!
Often we come across the existence of numbers, as they serve many functions in our lives, from counting the number of hours we sleep at night to the number of rounds we jog around the field track during a workout.
Numbers are a fundamental component of mathematics; they are categorised into several types according to their properties — odd, even, prime, composite numbers and more. We can apply numbers’ basic fundamental arithmetic operations and determine their nature, widening our minds to help us understand mathematics better. Along with numbers, we come across the exciting world of factors and multiples: HCF, LCM, and prime factorisation. They tell us how numbers are related and are an integral part of number theory and its analysis. So let’s begin the journey of numbers!
Euler’s Identity
Like in the short Sherlock Holmes story, A Scandal in Bohemia, Holmes meets and is bested by a lady named Irene Adler. While Holmes’s prevalent standpoint on the opposite sex is vague and ambivalent, he goes too far as to bless Ms. Adler with the honorific nickname ‘The Woman’, holding a photo of her as a reminder of how she outplayed him.
The equation which appears above is rightly highly recognised. Just like Ms. Adler, it has a claim to the substantial equation. The equation is also an omnipresent candidate in “What is the most elegant equation in Mathematics?” beauty parades. Indeed it has acquired an altogether inappropriate mystical quality. People feel it is ingrained with the meaning of the universe. The equation is, of course, critical, but not for esoteric reasons.
Euler’s Identity is expressed in math as the most stunning equation. The equation was first presented by Leonhard Euler, a renowned and prominent Swiss mathematician with an immense quantity of contributions to mathematics. Euler was accountable for much of the modern mathematical terminology and notation we all come to comprehend today.
To give you an idea, Euler widespread the Greek letter pi (π) to denote the circle’s circumference to its diameter and was arguably the first to utilise the notation of a function f(x). Though without a doubt, his most notable contribution was the Euler’s identity formula.
Keith J. Devlin, a 20th-century British mathematician and famous science writer on Euler’s identity formula, described it as “Like a Shakespearean sonnet that captures the very essence of love, or a painting that brings out the beauty of the human form that is far more than just skin deep, Euler’s equation reaches into the very depths of existence.”
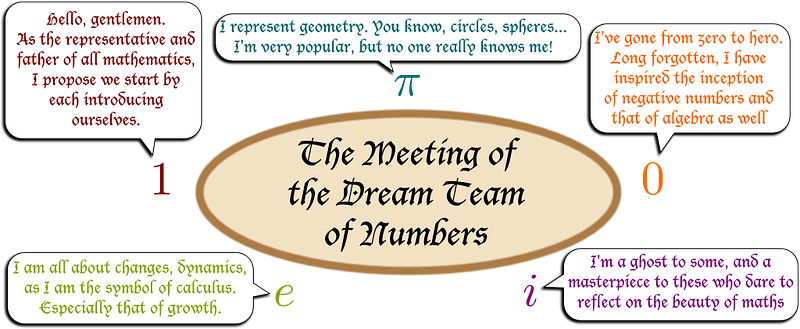
One reason that 𝑒𝑖𝜋+1=0 is such a beautiful equation is that it contains the five most essential constants in mathematics; 𝑒, 𝑖, 𝜋, 1 𝑎𝑛𝑑 0, and it also combines the basic operations of addition, multiplication, and exponentiation! It is astounding that such a formula does this. A study showed that Euler’s identity is so beautiful that it excites the same brain areas as a great piece of music or art would! To further understand this profound formula, we need to break down each part of the equation.
One is the first number to make it into the dream team of numbers, and historically, it is the first number invented, making it the father of all mathematics. Surprisingly, the second number to join the team is not 0, but 𝜋. 𝜋 is the most well-known irrational number found in so many math and science equations that it would astound you. Pi first appeared in antiquity, at least several millennia before our era, as the area of a disc of radius 1. It can be computed as an infinite expression, as shown below:
𝑒, on the other hand, is not entirely as well-known, but its importance is virtually equal to that of 𝜋. 𝑒 is created by Euler and named Euler’s number.
The main reason Euler presented this number was to elaborate on the natural phenomenon of 100% continuous growth. Imagine you put 1 dollar in your bank, and they tell you you’ll win a 100% interest rate every year. Now because they are crooks, they’ll only compute your interest rate at the end of the year. Thus you’ll get one more dollar and obtain a total of 2 dollars. 𝑒, as we know, has a value of 𝑒 ~ 2.718, so in reality, you’ll be making a 100% continuous growth of 2.718 dollars every end of the year.
Lastly, 𝑖 is the somewhat infamous ‘imaginary’ number defined as the square root of −1. While it is not an actual number and cannot be quantified on the number line; imaginary numbers are “real” in the sense that they exist and are used in math. Imaginary numbers, also represented as complex numbers, are used in real-life applications, such as electricity, as well as quadratic equations. In quadratic planes, imaginary numbers appear in equations that don’t touch the x-axis. Imaginary numbers become particularly useful in advanced calculus. It’s unlikely that any math equation will surpass this one in its beauty.
To conclude, all the numbers students usually hate, that annoying imaginary 𝑖; 𝜋, the circumference-to-diameter ratio that can never entirely be converted to dollars and cents; 𝑒, the amount in your bank account if you receive 100% interest, continuously compounded for a year on a deposit of $1 are all combined in one equation. It doesn’t make sense how could they all possibly have anything in common? This is mind-blowing! And it explains why Euler’s identity is often regarded as a feat of mathematics.
Perhaps this makes mathematics so uniquely interesting compared to other areas due to its surprising and unforeseen twists and turns. A vast amount of our understanding is due to a few distinguished mathematicians such as Newton, Euler, Gauss, or Riemann, whom have contributed something to human knowledge even more lasting than great literature since mathematics is independent of language.
Mathematics is an intrinsic part of the problem-solving, investigation, testing, design, and analysis work undertaken by people daily. It enables us to draw value from the data by exploring its patterns and estimating the confidence that might reasonably be put in the inferences drawn. A fascinating aspect of applying mathematics in the industry is that, in the practical environment, no particular solution ever seems directly applicable. Instead, the principles of past findings must be understood as a basis for drawing out different solutions, thereby enhancing and developing new theories to fit the real world. Having a solid understanding of the principles behind the known approach and the basis on which it has been created, researchers and graduate practitioners need to extend and apply that theory appropriately in the practical environment.
[Written by: Haris Ikhwan. Edited by: Teoh Jin]